Find the Solution to the Following Inequality 6x-5 7x 4

Related Pages
Solving Equations
Algebraic Expressions
More Algebra Lessons

The following figure shows how to solve two-step inequalities. Scroll down the page for more examples and solutions.

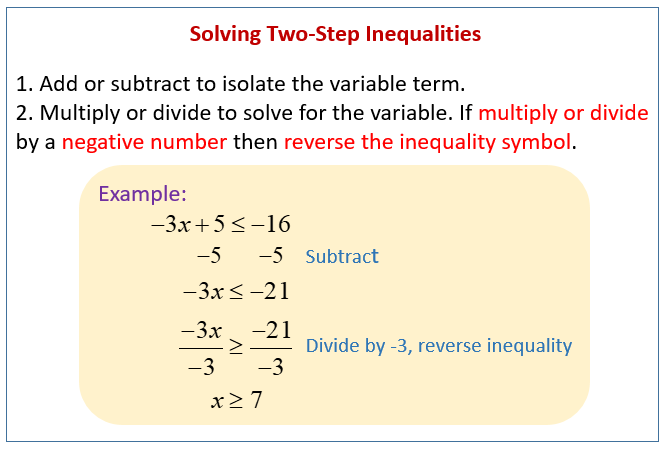
The rules for solving inequalities are similar to those for solving linear equations. However, there is one exception when multiplying or dividing by a negative number.

To solve an inequality, we can:

• Add the same number to both sides.
• Subtract the same number from both sides.
• Multiply both sides by the same positive number.
• Divide both sides by the same positive number.
• Multiply both sides by the same negative number and reverse the sign.
• Divide both sides by the same negative number and reverse the sign.

Inequalities Of The Form "x + a > b" or "x + a < b"

Example:
Solve x + 7 < 15

Solution:
x + 7 < 15
x + 7 – 7 < 15 – 7
x < 8

Inequalities Of The Form "x – a < b" or "x – a > b"

Example:
Solve x – 6 > 14

Solution:
x – 6 > 14
x – 6+ 6 > 14 + 6
x > 20

Example:
Solve the inequality x – 3 + 2 < 10

Solution:
x – 3 + 2 < 10
x – 1 < 10
x – 1 + 1 < 10 + 1
x < 11

Inequalities Of The Form "a – x < b" or "a – x > b"

Example:
Solve the inequality 7 – x < 9

Solution:
7 – x < 9
7 – x – 7 < 9 – 7
– x < 2
x > –2 (remember to reverse the symbol when multiplying by –1)

Example:
Solve the inequality 12 > 18 – y

Solution:
12 > 18 – y
18 – y < 12
18 – y – 18 < 12 –18
– y < –6
y > 6 (remember to reverse the symbol when multiplying by –1)

Inequalities Of The Form " < b" or " > b"

Example:
Solve > 3

Solution:
> 3
× 5 > 3 × 5

x > 15

Example:
Solve

Solution:

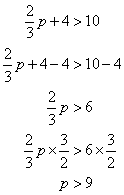
Solving Linear Inequalities With Like Terms

If an equation has like terms, we simplify the equation and then solve it. We do the same when solving inequalities with like terms.

Example:
Evaluate 3x – 8 + 2x < 12

Solution:
3x – 8 + 2x < 12
3x + 2x < 12 + 8
5x < 20
x < 4

Example:
Evaluate 6x – 8 > x + 7

Solution:
6x – 8 > x + 7
6x – x > 7 + 8
5x > 15
x > 3

Example:
Evaluate 2(8 – p) ≤ 3(p + 7)

Solution:
2(8 – p) ≤ 3(p + 7)
16 – 2p ≤ 3p + 21
16 – 21 ≤ 3p + 2p
–5 ≤ 5p
–1 ≤ p
p ≥ –1 (a < b is equivalent to b > a)

An Introduction To Solving Inequalities

• Show Video Lesson

Solving One-Step Linear Inequalities In One Variable

The solutions to linear inequalities can be expressed several ways: using inequalities, using a graph, or using interval notation.

The steps to solve linear inequalities are the same as linear equations, except if you multiply or divide by a negative when solving for the variable, you must reverse the inequality symbol.

Example:
Solve. Express the solution as an inequality, graph and interval notation.
x + 4 > 7
-2x > 8
x/-2 > -1
x - 9 ≥ -12
7x > -7
x - 9 ≤ -12

• Show Video Lesson

Solving Two-Step Linear Inequalities In One Variable

Example:
Solve. Express the solution as an inequality, graph and interval notation.
3x + 4 ≥ 10
-2x - 1 > 9
10 ≥ -3x - 2
-8 > 5x + 12

• Show Video Lesson

Solving Linear Inequalities

Main rule to remember: If you multiply or divide by a negative number, the inequality flips direction.

Examples of how to solve linear inequalities are shown:

Example:
Solve:
3x - 6 > 8x - 7

• Show Video Lesson

Solving Inequalities

Students learn that when solving an inequality, such as -3x is less than 12, the goal is the same as when solving an equation: to get the variable by itself on one side.

Note that when multiplying or dividing both sides of an inequality by a negative number, the direction of the inequality sign must be switched.

For example, to solve -3x is less than 12, divide both sides by -3, to get x is greater than -4.

And when graphing an inequality on a number line, less than or greater than is shown with an open dot, and less than or equal to or greater than or equal to is shown with a closed dot.

• Show Video Lesson

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.

Find the Solution to the Following Inequality 6x-5 7x 4

Source: https://www.onlinemathlearning.com/solving-inequalities.html

Share :